VBTutorial3

<< Return to the program

How to modify this page :

• first : log in (top right of this page) ;
• click on [edit] (far right) to edit a section of the page ;
• write your text directly in the wiki page, and click on the "Save page" button (bottom left) to save your modifications

Pictures : how to insert a picture in your text

See also this page for an introduction to the basics of the wiki syntax

State correlation diagrams

Remarks

>> Initial remarks for tutors

Exercices

Exercice 1 (paper exercice) : Conical intersection in H₃^• radical

Consider three hydrogen atoms Ha, Hb, Hc, with respective atomic orbitals a, b and c, and the two VB structures ] and ] .

The Ha-Hb and Hb-Hc distances are equal.

1. By using the thumb rules recalled below, where squared overlap terms are neglected, derive the expression of the energies of R and P, and of the reduced Hamiltonian matrix element between R and P for the 3-orbital/3-electrons reacting system [Ha--Hb--Hc]•.
2. From the sign of this latter integral when θ > 60°, derive the expressions of the ground state Ψ^≠ and of the first excited state Ψ* of the H₃• system. One may drop the normalization constants for simplicity. What bonding scheme does the excited state represent ?
3. Show that the reduced Hamiltonian matrix element is largest in the collinear transition state geometry, and drops to zero in the equilateral triangular structure.
4. Show that R and P VB structures are degenerate in the equilateral triangular structure, and that Ψ≠ and Ψ* are also degenerate in this geometry.
5. We now extend the above conclusions to the allyl radical. What are the bonding schemes corresponding to the ground state and first excited state ? What geometrical distortion would make these two states degenerate ? What would be the end product of a photochemical excitation of allyl radical to its first excited state ?

Appendix : Thumb rules for the calculations of effective Hamiltonian matrix elements between determinants.

1. Energy of a determinant D : 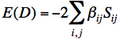] (if orbitals i and j have parallel spins)
2. Matrix element between determinants differing by spin inversion of two spin-orbitals :]

>> Answer

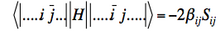Exercice 2 : computation of X—X + X. -> X. + X—X radical exchange VBSCD diagram for X=H,Li

1. Paper exercice :
   1. Beginning of ex. 6.3 from Sason & Philippe's book (first 3 lines)
   2. Exercice 6.5 from Sason & Philippe's book
   3. Exercice 6.6 questions a),b) and c)
   4. Exercice 6.6 question d)
   5. Exercice 6.6 question e)
2. Computer exercise :

idea : Compute VBSCD diagrams for X—X + X. -> X. + X—X X=H, Li at VBSCF then VBCI level. To be written...

>> Answer

Exercice 3 : Computation of state correlation Diagrams for a 3 centers / 4 electrons system

Sason's remark :

" F(-) + H-F example is not good by itself, unless you also do F• + H-F - showing that in one case you have an intermediate FHF(-) and in the other case you have a high barrier.

If we just want to do one case of 4-electron/3-center reaction, we should use Cl(-) + CH3Cl.

The audience will appreciate a more chemical example, which is Cl(-) + CH3Cl. "

Benoît's proposition :

1. Paper exercice :
   1. Exercice 6.12 question a) (from Sason & Philippe's book)
   2. Exercice 6.12 question b)
2. Computer exercise :

idea : Compute VBSCD diagrams for Cl(-) + CH3Cl -> ClCH3 + Cl(-), at D-BOVB levels, first in gas phase then using VB(PCM)... Which basis set should we use : 6-31+G*. As this is an anion we should add a set of diffuse functions, but then there may be trouble with BOVB... Check first that everything is fine at BOVB level (no instability)...