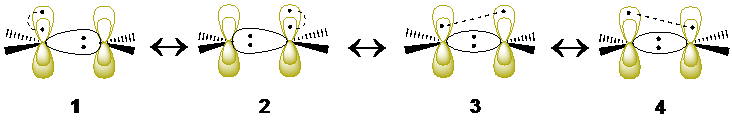Abstract pool hdr
Abstracts
Example: John Smith
University
Title
Text
[1] Popelier, P. L. A.; Brémond, É. A. G. Int.J.Quant.Chem. 2009, 109, 2542.
Vincent Tognetti, Laurent Joubert
Normandy Univ., COBRA UMR 6014 & FR 3038, Université de Rouen, INSA Rouen, CNRS, 1 rue Tesniere 76821 Mont St Aignan, Cedex, France.
vincent.tognetti@univ-rouen.fr
The many faces of halogen bond
In this talk, I will review part of our recent works dealing with halogen bonds[1] (ubiquitous in biology and material science), with a particular emphasis on interpretative tools based on the electron density.
In particular, conceptual DFT[2] will be invoked to characterize the electrophilicity of the halogen atoms through the usual or state-specific dual descriptors[3] and their partition in real space into reactive domains.[4] This arsenal will be shown to afford an explanation for the bond formation that is complementary to the pure electrostatic one embodied in the so-called σ-hole paradigm,[5] paradigm that we also recently revisited.[6]
In addition, the interactions at work once the complex is formed (while conceptual DFT focuses on the intrinsic properties of the fragments before they interact) will be analyzed in details using Pendás’ interacting quantum atoms scheme[7] within Bader’s atoms-in-molecules theory.[8] The role of secondary interactions, of covalency and dispersion will be particularly scrutinized, as well in intermolecular[9,10] as in intramolecular halogen bonds.[11-13]
[1] P. Metrangolo, G. Resnati, Cryst. Growth Des. 2012, 12, 5835
[2] P. Geerlings, F. De Proft, W. Lengenaeker, Chem. Rev. 2003, 103, 1793
[3] V. Tognetti, C. Morell, P.W. Ayers, L. Joubert, H. Chermette, Phys. Chem. Chem. Phys. 2013, 15, 14465
[4] V. Tognetti, C. Morell, L. Joubert, J. Comput. Chem. 2015, 36, 649
[5] P. Politzer, J.S. Murray, T. Clark, Phys. Chem. Chem. Phys. 2013, 15, 11178
[6] V. Tognetti, L. Joubert, Theor. Chem. Acc. 2015, 134, 90
[7] M.A. Blanco, A.M. Pendás, E. Francisco, J. Chem. Theory Comput. 2004, 1, 1096
[8] R.F.W. Bader, Atoms in Molecules: A Quantum Theory; Oxford University Press, New York, 1990
[9] O.A. Syzgantseva, V. Tognetti, L. Joubert, J. Phys. Chem. A 2013, 117, 8969
[10] V. Tognetti, L. Joubert, Challenges and Advances in Computational Chemistry and Physics, in press
[11] V. Tognetti, L. Joubert, J. Chem. Phys. 2013, 138, 024102
[12] M. Yahia-Ouahmed, V. Tognetti, L. Joubert, Comput. Theor. Chem. 2015, 1053, 254
[13] M. Yahia-Ouahmed, V. Tognetti, L. Joubert, in preparation
Dennis R. Salahub
University of Calgary, Department of Chemistry, Centre for Molecular Simulation, Institute for Quantum Science and Technology, Institute for Sustainable Energy, Environment and Economy, 2500 University Drive NW, Calgary, AB, Canada T2N 1N4
dennis.salahub@ucalgary.ca, xingliu@ucalgary.ca
Molybdenum carbide nanoparticles as catalysts for hydrogenation reactions, between clusters and the bulk
A gap exists in understanding heterogeneous catalysis between a cluster of a few atoms and a periodic slab model; reactions catalyzed by transition-metal-containing nanoparticles are still not well understood. In this presentation, we provide a multiscale modelling approach to study benzene hydrogenation on molybdenum carbide nanoparticles (MCNPs) in the process of in-situ heavy oil
upgrading. The QM DFTB method is coupled with an MM force field to yield a quantum mechanical/molecular mechanical (QM/MM) model describing the reactants, the nanoparticles and the surroundings. Umbrella sampling (US) was employed to calculate the free energy profiles for benzene hydrogenation in a model aromatic solvent under realistic conditions. Comparisons are made with the traditional methodologies; the results reveal new features of the metallic MCNPs. Under working conditions, rather than being rigid, they are very flexible due to the entropic contributions of the MCNPs and the solvent, which greatly affect the free energy profiles.
Mohamed Amaouch,a Julien Pilmé, a Nicolas Gallandb
aLaboratoire de Chimie Théorique Université Pierre et Marie Curie and CNRS. F-75005, Paris, France
bLaboratoire CEISAM, Université de Nantes, Nantes, France
The ELF and AIM topological analyses in the context of the quasirelativistic approach
There is currently a growing interest in using relativistic methods as it has become clear that the study of chemistry involving elements across the entire periodic table requires that relativistic effects are taken into account. The most rigorous approaches are based on the four-component (4c) formalism (Dirac equation) but several alternative two- component (2c) methods were proposed. The 2c methods are notably able to account for spin-orbit (SO) effects. However, the study of SO effects inside the chemical bond appears hindered mainly by the exclusive use of molecular orbital (MO) theory and the related Mulliken populations. Alternative strategies can be proposed to analyze the breaking and the formation of bonds between atoms : atoms in molecules theory (AIM) which relies on the properties (topology) of the total electron density when atoms interact or the Electron Localization Function (ELF) which is a signature of the electronic pairs distribution [1, 2, 3].In this context, an original formulation of ELF and AIM descriptors and their topologies were extended to the framework of 2c quantum calculations [4]. Illustrations of the SOC effects on the bond representation will be given for selected astatine (At, Z=85) species.
[1] Axel D Becke and Kenneth E Edgecombe. The Journal of chemical physics, 92(9) :5397-5403, 1990.
[2] Bernard Silvi and Andreas Savin. Nature, 371(6499) :683–686, 1994.
[3] Richard FW Bader. Atoms in molecules, 1990.
[4] Julien Pilmé, Eric Renault, Tahra Ayed, Gilles Montavon, and Nicolas Galland. Journal of Chemical Theory and Computation, 8(9) :2985–2990, 2012.
Roberto A. Boto
1. Sorbonne Universités. Université Pierre et Marie Curie. Institut du Calcul et de la Simulation. 75005. Paris. France.
2. Laboratoire de Chimie Théorique Université Pierre et Marie Curie and CNRS. F-75005, Paris. France.
On the interpretation of the reduced density gradient
Non-covalent interactions (NCIs) play a key role in many areas of science, ranging from biochemistry to condensed matter. In this regard, the NCI index has been proposed as a new tool to identify and characterise weak interactions of various strengths as chemical intuitive reduced density gradient (RDG) isosurfaces, that reveal both stabilising(hydrogen bonding and van der Waals interactions) and destabilising interactions.[1] Beyond qualitative analysis, the integration of both volume and charge within NCI isosurfaces are in good agreement with the hydrogen-bonding potential-energy surfaces. [2] Recently Saleh et al. found a good linear correlation between stabilisation energies and kinetic energies integrated on the main NCI isosurfaces. [3] These results connect the topology of RDG with energetics. In the present work we analyse the origin of these correlations through a set of molecular systems representing covalent, ionic and non-covalent interactions. A chemically sound interpretation of RDG is also presented.
[1] R. Johnson, S. Keinan, P. Mori-Sanchez, J. Contreras-Garcia, A. J. Cohen, and W. Yang, “Revealing Noncovalent Interactions”, J. Am. Chem. Soc. 132 , 6498 (2010)
[2] J. Contreras-Garcia, E. R. Johnson, W. Yang, “Analysis of Hydrogen-Bond Interaction Potentials from the Electron Density: Integration of Noncovalent Interaction Regions”, J. Phys. Chem. A 115, 12983 (2011)
[3] G. Saleh, C. Gatti, L. L. Presti, “Energetics of non-covalent interactions from electron energy density distributions”. Comp. Theo. Chem., 1053, 53 (2015)
Frank de Proft
Eenheid Algemene Chemie (ALGC), Vrije Universiteit Brussel (VUB), Pleinlaan 2, B-1050 Brussels, Belgium.
Density Functional Theory Reactivity Indices: Theoretical Developments,
Application in Transition Metal Chemistry and Inverse Design of Molecules with
Optimal Reactivity Properties
Conceptual Density Functional Theory (sometimes also called DFT based reactivity theory or Chemical DFT) has proven to be an ideal framework for the introduction of chemical reactivity descriptors. These indices are defined as response functions of the energy E of the system with respect to either the number of electrons N, the external potential ν(r) or both. These definitions have afforded their non- empirical calculation and applications in many fields of chemistry have been performed.
In a first part of the talk, attention will be focused on the so-called linear response function (or polarizability kernel) defined as the second derivative of the energy with respect to the external potential. We will give an overview of the chemistry that can be extracted from this quantity: the kernel is shown to provide a measure of electron delocalization and a connection is made to the concept of aromaticity.
In a second part, an investigation will be presented of structural and electronic requirements of ligand non-innocent behavior in transition metal complexes.
Finally, we will highlight an application of the so-called inverse design methodology, using the reactivity indices to design molecules with optimal reactivity properties. More specifically, this approach will be used to construct radicals with unprecedented stabilities. The design of molecules with optimal properties is an important challenge in chemistry because of the astronomically large number of possible stable structures that are accessible in chemical space. The thiadiazinyl radical was chosen as case study of this new approach, and the optimized property of this compound was the intrinsic radical stability. The resulting optimal structure was found to be highly stable, intrinsically more so than other well-known stable radicals.
Henry Chermette,a Walid Lamine,a,b Christophe Morella
a Université de Lyon, Université Claude Bernard Lyon 1, Institut des Sciences Analytiques, UMR CNRS 5280, 69622 Villeurbanne Cedex, France
b Université de Tunis El Manar, Faculté des Sciences de Tunis, UR11ES19 Unité de recherche Physico-Chimie des Matériaux condensés, El-Manar II, 2092, Tunis, Tunisie
Ill-advised self-interaction contribution in modeling anionic attack in a reaction path.
The catalytic role of an anion such as iodide in the insertion of CO2 into an epoxide over a Zn complex is presented.
It is shown how the contribution of the iodide ion may be incorrectly modeled in a reaction path, and how the ill-advised self-interaction contribution may be approximately cured.
Philippe C. Hiberty, Wei Wu, Huaiyu Zhang, Benoît Braida, Sason Shaik
V state of ethylene: from myriads of MO-CI configurations to just four valence bond structures
The first singlet excited state of ethylene (so-called the V state) is a notoriously difficult test case that has necessitated elaborate strategies and extensive configuration interaction in the molecular orbital (MO) framework. By contrast, the description of this electronic state and its transition energy from the ground state (so-called the N state), becomes very simple with valence bond methods. It is shown that extremely compact wave functions, made of three VB structures for the N state and four structures for the V state (1-4 in the scheme below), provide an N→V transition energy of 8.01 eV, in good agreement with experiment (7.88 eV for the N→V transition energy estimated from experiments). Further improvement to 7.96/7.93 eV is achieved at the variational and diffusion Monte Carlo (MC) levels, using a Jastrow factor coupled with the same compact VB wave functions. Furthermore, the measure of the spatial extension of the V state wave function, 19.14 a02, is in the range of accepted values obtained by large-scale state-of-the-art MO-based methods. The σ response to the fluctuations of the π electrons in the V state, known to be a crucial feature of the V state, is taken into account using the breathing-orbital valence bond method (BOVB), which allows the VB structures to have different sets of orbitals, as is made apparent in 1 and 2. Further valence bond calculations in a larger space of configurations confirm the results of the minimal structure-set, yielding an N→V transition energy of 7.97 eV and a spatial extension of 19.16 a02 for the V state. Both types of valence bond calculations show that the V state of ethylene is not fully ionic as usually assumed, but involving also a symmetry-adapted combination of VB structures,3 and 4, each with asymmetric covalent π bonds. The latter VB structures have cumulated weights of at least 18-26%, and stabilize the V state by about 0.9 eV. It is further shown that these latter VB structures, rather than the commonly considered zwitterionic ones, are the ones responsible for the spatial extension of the V state, known to be ca. 50% larger than the V state.
Aurélien de la Lande
Laboratoire de Chimie Physique, Université Paris Sud, CNRS, Université Paris Saclay. 15, avenue Jean Perrin, 91405 Orsay, Cedex. France
Robust, basis-set independent method for the evaluation of charge-transfer energy in nonconvalent complexes
Separation of the energetic contribution of charge transfer to interaction energy in noncovalent complexes would provide important insight into the mechanisms of the interaction. However, the calculation of charge-transfer energy is not an easy task. It is not a physically well-defined term and the results might depend on how it is described in practice. Commonly, the charge transfer is defined in terms of molecular orbitals; in this framework, however, the charge transfer vanishes as the basis set size increases towards the complete basis set limit. This can be avoided by defining the charge transfer in terms of the spatial extent of the electron densities of the interacting molecules, but the schemes used so far do not reflect the actual electronic structure of each particular system and thus are not reliable. We propose a novel approach – spatial partitioning of the system which is based on a charge transfer-free reference state, namely superimposition of electron densities of the non-interacting fragments. We show that this method, employing constrained DFT for the calculation of the charge-transfer energy, yields reliable results and is robust with respect to the strength of the charge transfer, the basis set size and the DFT functional used. Because it is based on DFT, the method is applicable to rather large systems.
[1] Řezáč, J; de la Lande, A. J. Chem. Theor. Comput. 2015, 11, 528-537.
A. Martín Pendás
Universidad de Oviedo, Julian Claveria, 33006, Oviedo, Spain
Electron distribution functions from academic models: towards a real space Aufbau principle
Electron number distribution functions (EDFs) allow for the computation of the weights of
real space resonance structures. To obtain them we need a partition of
space into chemically meaningul regions, i.e. through QTAIM, ELF, Hirshfeld, or any other
exhaustive or fuzzy decomposition available in the literature.
With such a decomposition we may compute the probability of distributing the N electrons of a molecular system into the m regions in which we have divided space, in every possible way. EDFs provide valuable insight into chemical bonding, and here we show that they may be successfully approximated by very simple models, giving rise to an interesting interpretation of the standard Aufbau principle in real space. This is obtained from academic models of the wave functions of simple systems and a Mulliken-like condensation.
P. Reinhardt, P. Chaquin
LCT, UPMC, Paris, France
Realistic molecular frequencies from a simple atomic-density model
Based on the Hellmann-Feynman theorem we construct a simple model for calculating force constants in molecular, poly-nuclear frameworks. We show the vibrational frequencies for homonuclear diatomics are obtained with an astonishing precision, and for larger molecules domains of frequencies can be identified.