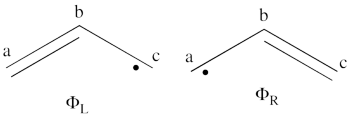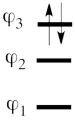Différences entre les versions de « VBTutorial2 »
| Ligne 506 : | Ligne 506 : | ||
== Exercise 4 : The allyl cation == | == Exercise 4 : The allyl cation == | ||
| − | + | ||
| − | + | {| class="collapsible collapsed wikitable" | |
| + | |- | ||
| + | !<big>'''Paper exercise'''</big> | ||
| + | |- | ||
| + | | | ||
# Structures of the allyl cation, and weights from Hückel theory : | # Structures of the allyl cation, and weights from Hückel theory : | ||
## Find a complete basis of covalent structures for the allyl cation. | ## Find a complete basis of covalent structures for the allyl cation. | ||
## What are the possible ionic structures for the allyl cation ? Based on your chemical knowledge, propose a selection subset of the most chemically meaningful covalent + ionic structures. | ## What are the possible ionic structures for the allyl cation ? Based on your chemical knowledge, propose a selection subset of the most chemically meaningful covalent + ionic structures. | ||
| − | ## Use the [http://www.hulis.free.fr/ HuLis] software to retrieve the Hückel MOs for allyl cation. Write a single-determinant MO wave function based on Hückel orbitals. Develop it into the basis of atomic orbitals, to get an expression in terms of VB structures. Compute by hand the weights of the different structures (neglecting all overlaps for simplicity). <br> <br> | + | ## Use the [http://www.hulis.free.fr/ HuLis] software to retrieve the Hückel MOs for allyl cation. Write a single-determinant MO wave function based on Hückel orbitals. Develop it into the basis of atomic orbitals, to get an expression in terms of VB structures. Compute by hand the weights of the different structures (neglecting all overlaps for simplicity). |
| − | < | + | |
| + | {| class="collapsible collapsed wikitable" | ||
| + | |- | ||
| + | !'''Answer''' | ||
| + | |- | ||
| + | | | ||
| + | ====''Structures of the allyl cation, and weights from Hückel theory: ''==== | ||
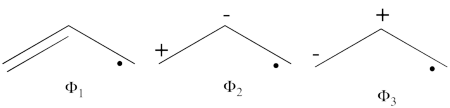
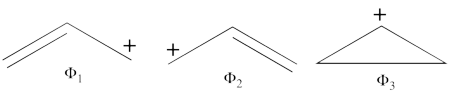
| + | # There are three covalent structures for the allyl cation as follows:<br> [[File:Allyl_CovCation-N1.png|450px]] <br> the third covalent structure is expeceted to be higher in energy than the other two structures and thus, contribute less. | ||
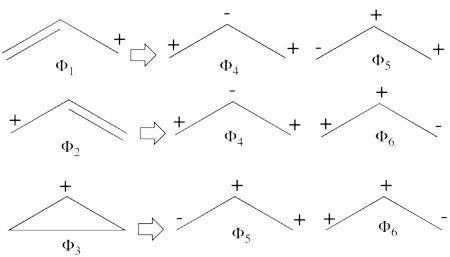
| + | #From each covalent structure we can get two ionic structures. Some of them are however common, leading to an overall of 3 different ionic structures (<math> \Phi_{\textrm{4}} </math>, <math> \Phi_{\textrm{5}} </math>, <math> \Phi_{\textrm{6}} </math>). <br> [[File:Allyl_IonCation-N1.png|450px]] <br> (<math> \Phi_{\textrm{5}} </math> and <math> \Phi_{\textrm{6}} </math>) are expected to be high in energy. Therefore, our selected subset will involve 4 strucutres, (<math> \Phi_1, \Phi_2, \Phi_3 </math> and <math> \Phi_{\textrm{4}} </math>). | ||
| + | #The MO orbitals in the Huckel approximation are: <br> <math> | ||
| + | \varphi_1 = \frac{1}{2}(p_1 + \sqrt{2}p_2 + p_3) </math> <br> <math> | ||
| + | \varphi_2 = \frac{\sqrt2}{2}(p_1 - p_3)</math> <br> <math> | ||
| + | \varphi_3 = \frac{1}{2}(p_1 - \sqrt{2}p_2 + p_3) </math> <br> A single-determinant MO wavefunction of allyl cation based on these Hückel orbitals would look as follows: <br> <math> | ||
| + | \psi_{H\ddot{u}ckel} = \vert \varphi_1\bar{\varphi_1}\vert = | ||
| + | \vert \frac{1}{2}(p_1 + \sqrt{2}p_2 + p_3)\overline{\frac{1}{2}(p_1 + \sqrt{2}p_2 + p_3)}\vert | ||
| + | </math> <br> Developing it into the basis atomic orbitals we get: <br> <math> | ||
| + | \psi_{H\ddot{u}ckel} = | ||
| + | \frac{1}{4}(\vert p_1 \overline p_1\vert + \sqrt{2}\vert p_1 \overline p_2\vert + \vert p_1 \overline p_3\vert + | ||
| + | \sqrt{2}\vert p_2 \overline p_1\vert + 2\vert p_2 \overline p_2\vert + \sqrt{2}\vert p_2 \overline p_3\vert + | ||
| + | \vert p_3 \overline p_1\vert + \sqrt{2}\vert p_3 \overline p_2\vert + \vert p_3 \overline p_3\vert) = | ||
| + | </math> <br> we will now reorder the components: <br> <math> | ||
| + | \psi_{H\ddot{u}ckel} = | ||
| + | \frac{1}{2}(\vert p_2\overline p_2\vert) + | ||
| + | \frac{1}{4}(\vert p_1\overline p_1\vert) + \frac{1}{4}(\vert p_3\overline p_3\vert) + | ||
| + | \frac{\sqrt2}{4}(\vert p_1\overline p_2\vert) - (\vert \overline p_1 p_2\vert) + | ||
| + | \frac{\sqrt2}{4}(\vert p_2\overline p_3\vert) - (\vert \overline p_3 p_2\vert) + | ||
| + | \frac{1}{4}(\vert p_1\overline p_3\vert) - (\vert \overline p_1 p_3\vert) | ||
| + | </math> <br> <math> | ||
| + | \psi_{H\ddot{u}ckel} = | ||
| + | \frac{1}{2}\Phi_4 + | ||
| + | \frac{1}{4}(\Phi_5 + \Phi_6) + | ||
| + | \frac{1}{2}(\Phi_1 + \Phi_2) + | ||
| + | \frac{\sqrt2}{4}\Phi_3 | ||
| + | </math> <br> The weights while neglecting overlap are therefore:<br> <math> | ||
| + | \psi_{H\ddot{u}ckel} = | ||
| + | 25%\Phi_4 + | ||
| + | 6.25%\Phi_5 + 6.25%\Phi_6 + | ||
| + | 25%\Phi_1 + 25%\Phi_2 + | ||
| + | 12.5%\Phi_3 | ||
| + | </math><br> | ||
| + | |} | ||
| + | |||
| + | |} | ||
| + | |||
| + | {| class="collapsible collapsed wikitable" | ||
| + | |- | ||
| + | !<big>'''Computer Exercise'''</big> | ||
| + | |- | ||
| + | | | ||
# Computation of allyl cation energies and weights : | # Computation of allyl cation energies and weights : | ||
## Compute a VBSCF wave function for allyl cation (6-31G basis set) using your selected set of structures (questions 1.3 and 2.2). Compute a VBSCF wave function including the complete set of VB structures, using the '' "str=full" '' keyword. Compare the weights and energies for both wave functions to validate your selection of structures. | ## Compute a VBSCF wave function for allyl cation (6-31G basis set) using your selected set of structures (questions 1.3 and 2.2). Compute a VBSCF wave function including the complete set of VB structures, using the '' "str=full" '' keyword. Compare the weights and energies for both wave functions to validate your selection of structures. | ||
| Ligne 520 : | Ligne 572 : | ||
## We want to build a wave-function corresponding to only one ''Lewis'' structures for the allyl cation. To do so, we will include in the wave-function only one covalent structure, and the ionic structures associated to this covalent bond. Propose a selection of ''VB'' structures which would describe one ''Lewis'' structure for the allyl cation. | ## We want to build a wave-function corresponding to only one ''Lewis'' structures for the allyl cation. To do so, we will include in the wave-function only one covalent structure, and the ionic structures associated to this covalent bond. Propose a selection of ''VB'' structures which would describe one ''Lewis'' structure for the allyl cation. | ||
## Compute this wave function at VBSCF then BOVB levels. Deduce what is the resonance energy of the allyl cation at the BOVB level. | ## Compute this wave function at VBSCF then BOVB levels. Deduce what is the resonance energy of the allyl cation at the BOVB level. | ||
| − | <br> | + | |} |
| + | |||
| + | {| class="collapsible collapsed wikitable" | ||
| + | |- | ||
| + | !'''Anwser''' | ||
| + | |- | ||
| + | | | ||
| + | ====''Computation of allyl cation energies and weights''==== | ||
| + | |||
| + | # The total energy of the VB wave function with selected set of structures for allyl cation is summarized in the following Table: | ||
| + | |||
| + | <center> | ||
| + | {| border="1" | ||
| + | |+ VB energies of the allyl cation | ||
| + | ! scope="col" | Structures & Method | ||
| + | ! scope="col" | Total Energy (au) | ||
| + | |- | ||
| + | ! scope="row" | 3 cov + 1 ionic (VBSCF) | ||
| + | | -116.10652003 | ||
| + | |- | ||
| + | ! scope="row" | Str=full (VBSCF) | ||
| + | | -116.10767709 | ||
| + | |- | ||
| + | ! scope="row" | 3 cov + 1 ionic (BOVB) | ||
| + | | -116.14182494 | ||
| + | |} | ||
| + | </center><br>The energy difference is -0.72 kcal/mol within the VBSCF. Comparison of VBSCF with BOVB both when only using the selected subset, one gets a difference of -22.15 kcal/mol<br><center> | ||
| + | {| border="1" | ||
| + | |+ Weights of the VB structures of the allyl cation | ||
| + | ! scope="col" | | ||
| + | ! scope="col" | Str=full (VBSCF) | ||
| + | ! scope="col" | 3 cov + 1 ion (VBSCF) | ||
| + | ! scope="col" | 3 cov + 1 ion (BOVB) | ||
| + | |- | ||
| + | ! scope="row" |<math> \phi_1 </math> 1:10 11 12 | ||
| + | | 0.31366 || 0.31915 || 0.30595 | ||
| + | |- | ||
| + | ! scope="row" | <math> \phi_2 </math> 1:10 12 13 | ||
| + | | 0.31366 || 0.31915 || 0.30595 | ||
| + | |- | ||
| + | ! scope="row" | <math> \phi_3 </math> 1:10 11 13 | ||
| + | | 0.19911 || 0.19723 || 0.20652 | ||
| + | |- | ||
| + | ! scope="row" | <math> \phi_4 </math> 1:10 12 12 | ||
| + | | 0.15690 || 0.16448 || 0.18158 | ||
| + | |- | ||
| + | ! scope="row" | <math> \phi_5 </math> 1:10 11 11 | ||
| + | | 0.00833 | ||
| + | |- | ||
| + | ! scope="row" | <math> \phi_6 </math> 1:10 13 13 | ||
| + | | 0.00833 | ||
| + | |}</center><br>when comparing 3 cov + 1 ion with Str=full we see that the weights of the last two structures in the second calculation (the more comprehensive calculation) are indeed very small. | ||
| + | <br> | ||
| + | |||
| + | ===='' Resonance energies of allyl cation ''==== | ||
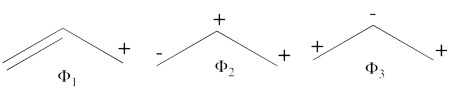
| + | #The following are the three possible structures of allyl cation which describe one Lewis structure:<br>[[File:Allyl_Cation_Lewis.png|450px]]<br> | ||
| + | #The total energy of the VB wave function of the Lewis structure of allyl cation | ||
| + | <br> | ||
| + | {| border="1" | ||
| + | |+ Total energies and weights of the Lewis structure of the allyl cation | ||
| + | ! scope="col" | | ||
| + | ! scope="col" | VBSCF | ||
| + | ! scope="col" | BOVB | ||
| + | |- | ||
| + | ! scope="row" | Total energy (au) | ||
| + | | -116.045854 || -116.0645573 | ||
| + | |- | ||
| + | ! scope="row" | weight of <math> \Phi_1 </math> | ||
| + | | 0.74056 || 0.66590 | ||
| + | |- | ||
| + | ! scope="row" | weight of <math> \Phi_2 </math> | ||
| + | | 0.03291 || 0.05006 | ||
| + | |- | ||
| + | ! scope="row" | weight of <math>\Phi_3 </math> | ||
| + | | 0.22654 || 0.28404 | ||
| + | |||
| + | |} | ||
| + | <br> | ||
| + | * At the BOVB level the resonance energy (RE) of the allyl cation is a difference between the total energy of the allyl radical with full set of the structure and the total energy of the Lewis structure only. RE is 48.49 kcal/mol. | ||
| + | |} | ||
| − | |||
|} | |} | ||
[[General_guidelines_for_BOVB_calculations| >> general guidelines for BOVB calculations]] | [[General_guidelines_for_BOVB_calculations| >> general guidelines for BOVB calculations]] | ||
Version du 12 juillet 2012 à 10:12
VB applications on PI systems
In all the following exercises, <math>\pi</math> the system will be taken as active, and the <math>\sigma</math> system as inactive. In all VB calculations, the <math>\sigma</math> orbitals shall be described by MOs delocalized onto the whole molecule.
| Main exercises | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
Exercise 1 : The allyl radical
Exercise 2 : Radical character of ozone
|
| Optional Exercises - Homework | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
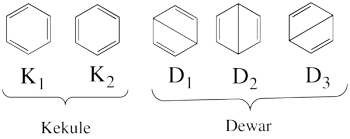
Exercise 3 : Resonance energy of Benzene
Exercise 4 : The allyl cation
|